top of page

This page should give you an idea on finding area for circles and solving complicated integrals that require trigonometric substitution.
(Be warned, immense and complicated math talk ahead.)
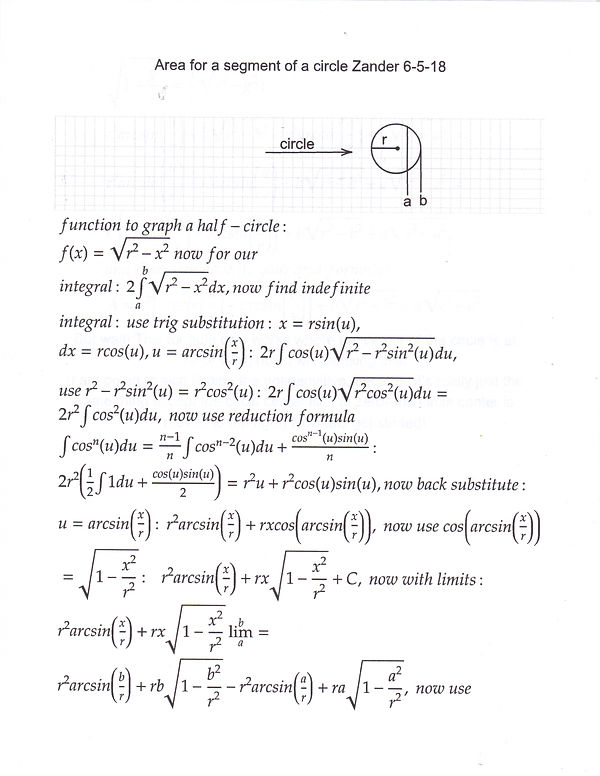


My friend actually did this without calculus. He used trigonometry to do it, which is quite impressive.
Here I simply moved the circle on the graph one radius length to the right. So the half circle is in the upper right quadrant in the graph. After that I did the same process as before.
bottom of page